Wave Walker DSP

DSP Algorithms for RF Systems

Trending

Buy the Book!

DSP for Beginners: Simple Explanations for Complex Numbers! The second edition includes a new chapter on complex sinusoids.

bookCover2Ed
examples1
examples2
eulersFormula
radians
negativeFrequency
adding
posNegAngles
complexSinusoidPhase
Easy Guide to Logarithms and Decibels
July 20, 2022

Table of Contents

Introduction

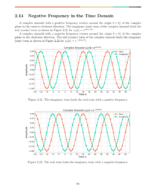
Logarithms are confusing! This blog explains what logarithms are, how they are used, and gives two easy equations to convert between decibels and linear units.

Check out these other blogs on DSP math:

What's a Base?

A number can be represented by a base raised to an exponent. For example, the number 100 can be written as

(1)   \begin{equation*}100 = 10^2.\end{equation*}

The number 10 is the base and the exponent is 2. Here are more examples:

(2)   \begin{equation*}1000 = 10^3,\end{equation*}

(3)   \begin{equation*}\pi \approx 10^{0.49715}.\end{equation*}

The base can be changed to other numbers. For example, base 2:

(4)   \begin{equation*}16 = 2^4,\end{equation*}

(5)   \begin{equation*}6.7 \approx 2^{2.7442}.\end{equation*}

The base can also be Euler’s number e,

(6)   \begin{equation*}10 \approx e^{2.3026},\end{equation*}

(7)   \begin{equation*}45.1 \approx e^{3.8089}.\end{equation*}

Why Logarithms?

The base of a logarithm is written as a subscript. For example, a base 10 logarithm is written as \text{log}_{10}\left( \cdot \right), a base 2 logarithm is written as \text{log}_{2}\left( \cdot \right), and a natural logarithm may be written as \text{log}_{e}\left( \cdot \right) or \text{log}\left( \cdot \right).

Logarithms are used to represent numbers by their exponent. Logarithms act as if a number is represented by a base raised to an exponent, and then they calculate that exponent.

From (1), (2), (5) and (7),

(8)   \begin{equation*}\text{log}_{10}\left( 100 \right) = \text{log}_{10}\left( 10^2 \right) = 2,\end{equation*}

(9)   \begin{equation*}\text{log}_{10}\left( 1000 \right) = \text{log}_{10}\left( 10^3 \right) = 3,\end{equation*}

(10)   \begin{equation*}\text{log}_{2}\left( 6.7 \right) = \text{log}_{2}\left( 2^{2.7442} \right) = 2.7442,\end{equation*}

(11)   \begin{equation*}\text{log}_{e}\left( 45.1 \right) = \text{log}_{e}\left( e^{3.8089} \right) = 3.8089.\end{equation*}

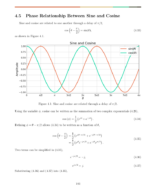
Linear to Decibels

Engineers commonly use 10\text{log}_{10} (\cdot) to transform linear numbers into units of decibels,

(12)   \begin{equation*}x_{dB} = 10\text{log}_{10}( x )\end{equation*}

which can then be transformed back into linear units through

(13)   \begin{equation*}x = 10^{x_{dB}/10}.\end{equation*}

Here are some examples using (12) and (13).

Example 1:

(14)   \begin{equation*}10\text{log}_{10}\left( 10 \right) = 10,\end{equation*}

(15)   \begin{equation*}10^{10/10} = 10.\end{equation*}

Example 2:

(16)   \begin{equation*}10\text{log}_{10}\left( 100 \right) = 20,\end{equation*}

(17)   \begin{equation*}10^{20/10} = 10^{2} = 100.\end{equation*}

Example 3:

(18)   \begin{equation*}10\text{log}_{10}\left( 0.5 \right) = -3.01,\end{equation*}

(19)   \begin{equation*}10^{-3.01/10} = 10^{-0.301} \approx 0.5.\end{equation*}

Example 4:

(20)   \begin{equation*}10\text{log}_{10}\left( 2\pi \right) = 7.9818,\end{equation*}

(21)   \begin{equation*}10^{7.8918/10} = 10^{0.79818} \approx 2 \pi.\end{equation*}

Conclusion

Numbers can be represented by a base number raised to an exponent. Logarithms are useful in representing the exponents of base numbers. Engineers use 10log10() to convert linear units into decibels.

Check out these other blogs on DSP math:

Leave a Reply

For everything there is a season, and a time for every matter under heaven. A time to cast away stones, and a time to gather stones together. A time to embrace, and a time to refrain from embracing. Ecclesiastes 3:1,5
The earth was without form and void, and darkness was over the face of the deep. And the Spirit of God was hovering over the face of the waters. Genesis 1:2
Behold, I am toward God as you are; I too was pinched off from a piece of clay. Job 33:6
Enter His gates with thanksgiving, and His courts with praise! Give thanks to Him; bless His name! Psalm 100:4
Lift up your hands to the holy place and bless the Lord! Psalm 134:2
Blessed is the man who trusts in the Lord, whose trust is the Lord. He is like a tree planted by water, that sends out its roots by the stream, and does not fear when heat comes, for its leaves remain green, and is not anxious in the year of drought, for it does not cease to bear fruit. Jeremiah 17:7-8
He said to him, “You shall love the Lord your God with all your heart and with all your soul and with all your mind. This is the great and first commandment. And a second is like it: You shall love your neighbor as yourself. On these two commandments depend all the Law and the Prophets.” Matthew 22:37-39
Then He said to me, “Prophesy over these bones, and say to them, O dry bones, hear the word of the Lord. Thus says the Lord God to these bones: Behold, I will cause breath to enter you, and you shall live." Ezekiel 37:4-5
Riches do not profit in the day of wrath, but righteousness delivers from death. Proverbs 11:4
The angel of the Lord appeared to him in a flame of fire out of the midst of a bush. He looked, and behold, the bush was burning, yet it was not consumed. And Moses said, “I will turn aside to see this great sight, why the bush is not burned.” When the Lord saw that he turned aside to see, God called to him out of the bush, “Moses, Moses!” And he said, “Here I am.” Exodus 3:2-3
Daniel answered and said: “Blessed be the name of God forever and ever, to whom belong wisdom and might. He changes times and seasons; He removes kings and sets up kings; He gives wisdom to the wise and knowledge to those who have understanding." Daniel 2:20-21
Now the Lord is the Spirit, and where the Spirit of the Lord is, there is freedom. 2 Corinthians 3:17
Previous slide
Next slide

This website participates in the Amazon Associates program. As an Amazon Associate I earn from qualifying purchases.

© 2021-2024 Wave Walker DSP