New WWDSP Book For Sale!
March 1, 2025
Trending
Install and Run LaTeX on macOS - Introduction to Interpolation and Upsampling
- Designing FIR Filter Gain
- What is Power Spectral Density (PSD)?
- Building a PSK and QAM Modulator in Python
- How to Write a Technical Paper
- Using Euler’s Formula to Derive Sine and Cosine
- Phase, Frequency and Negative Frequency in Complex Sinusoids
- Complex Frequency Shifting in Discrete Time
- How to Create Additive White Gaussian Noise (AWGN)
Recent Blogs
Apollo 13 and Engineering Operations
February 1, 2025
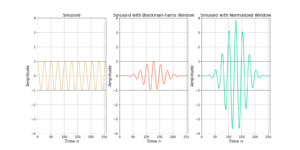
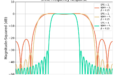
Windowing Function Gain Normalization
January 1, 2025
Should I Get a Masters Degree?
December 1, 2024
What DSP Classes Should I Take?
November 1, 2024
How To Install GNU Radio Source Code
October 1, 2024
How to Travel Well At the Airport
September 1, 2024
Celebrating Three Years of WWDSP!
August 28, 2024
How to Count in Binary Numbers
August 1, 2024
![A BPSK signal s[n], real Gaussian noise w[n], and the received signal x[n] = s[n] + w[n] for SNR = 20 dB](https://www.wavewalkerdsp.com/wp-content/uploads/2023/12/HowToComputeSignalToNoiseRatioSNRInSimulation_signalNoiseTimeDomain-300x240.png)
Buy the Book!
The third edition of DSP for Beginners: Simple Explanations for Complex Numbers! Includes a new chapter on sampling.
